Unit 1 Practice Quiz

  1. A swimming pool that holds a maximum of 5000 cubic feet will hold          imperial gallons. Calculate the value to the nearest hundredth.
  2. A gas tank of a car has a volume of 3.62 cubic feet. In order to fill up the tank, it would take          US gallons. Calculate the value to the nearest hundredth.
  3. You are given a 5 US gallon pail to fill with water.  If you fill it up to the top it will weigh          pounds. Calculate the value to the nearest hundredth.
  4. 4 Litres of water weighs 4 pounds. True or false?
  5. A 4 litre milk carton has          imperial gallons. Calculate the value to the nearest hundredth.

Answers

  1. 31,200 (imperial gallons)
  2. 27.10 or 27.08 (US gallons)
  3. 41.65 (pounds)
  4. False
  5. 0.88 (imperial gallons)

Unit 2 Practice Quiz

  1. “Force over a defined area” is the definition of:
    1. Pressure
    2. Force
    3. PSI
    4. Newton
  2. The pressure (in psi) at the base of a piping system filled with water with a height of 17 feet would be          psi. Calculate the value to the nearest hundredth.
  3. A piping system runs through three storeys of a building. Each story is 9 feet high. Water is filled to the top of the third storey. What is the total force at the base of the piping system given that the pipe has a diameter of 4 inches?Calculate the value to the nearest hundredth.
  4. The total force acting at the base of a swimming pool which measures 40 feet by 20 feet and haas a depth of 5 feet is           pounds. Calculate the value to the nearest hundredth.
  5. A piping system requires that a 6 inch gate valve be installed.  It is determined that the system pressure is 120 psi and this pressure will be applied to the gate valve when it is closed.  The total force acting on the gate valve is          pounds. Calculate the value to the nearest hundredth.

Answers

  1. Pressure
  2. 75.78 (psi)
  3. 146.90 pounds
  4. 249,408 (pounds)
  5. 3392.93 or 3393 (pounds)

Unit 3 Practice Quiz

  1. A pipe is run at a grade of 1/8” /ft. The Pipe is run a distance of 324 feet. The total fall on the pipe is          inches. Calculate the value to the nearest hundredth.
  2. A storm drain runs 100 feet. The grade on the storm drain is 1/4″ /ft. The total fall of the storm drain is          inches. Calculate to the nearest inch.
  3. A 157 meter 6” drainage line required to slope at a grade of 1/100. The total fall of the line is          metres. Calculate the value to the nearest hundredth.
  4. A pipe runs a distance of 500 feet. During the run of the pipe it drops 3 feet. The grade of the pipe (expressed as a percent) is         %. Calculate to the nearest tenth.
  5. A branch in a drainage system is 8 feet long. The grade on the pipe is 2.08%. The total fall on the branch (in inches) is          inches. Calculate to the nearest hundredth.

Answers

  1. 40.5 (inches)
  2. 25 (inches)
  3. 1.57 (metres)
  4. 0.6 (%)
  5. 1.99 or 2 (inches)

Unit 4 Practice Quiz

Use the image of the triangle below to answer the following questions:

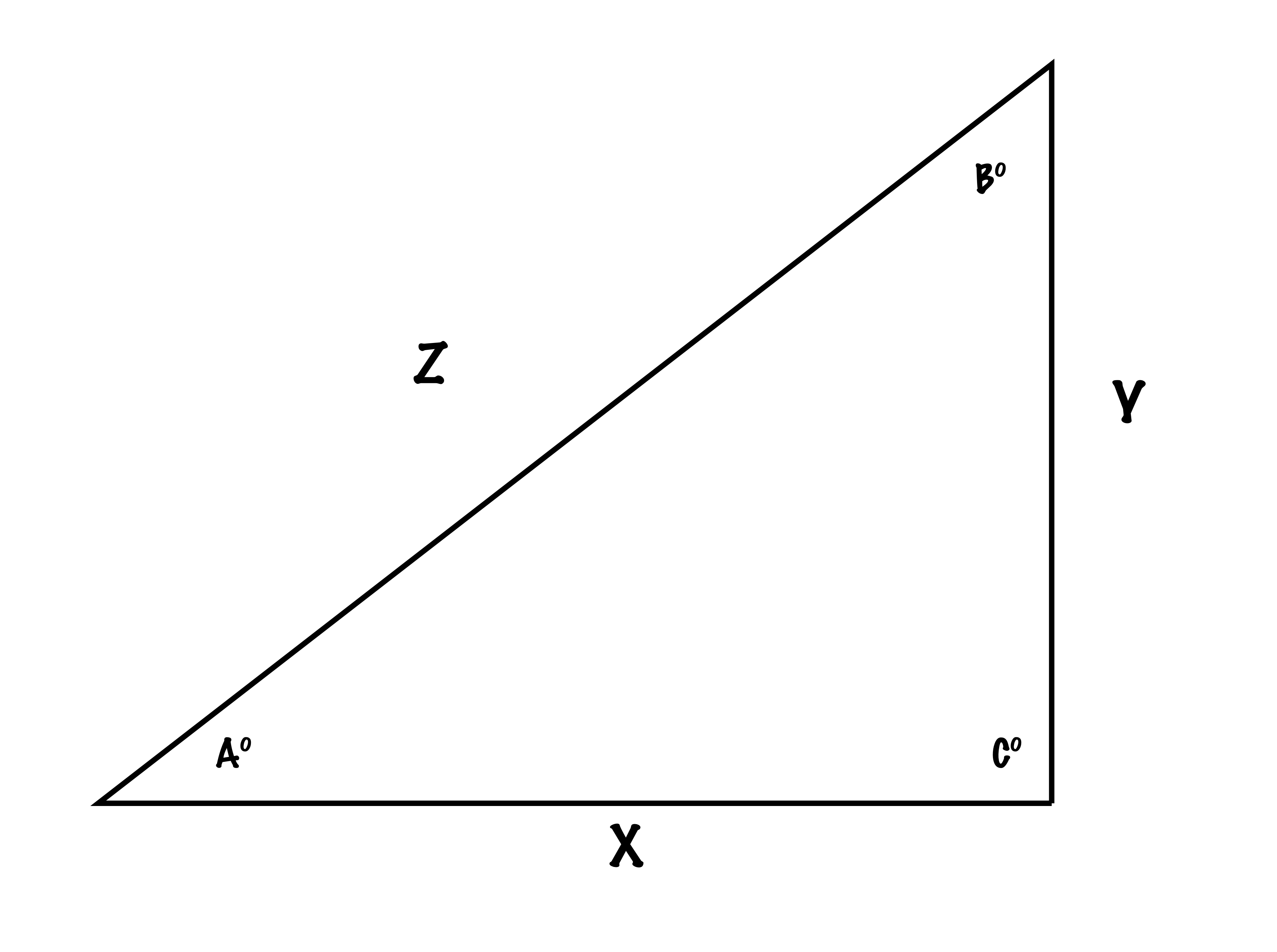
A right triangle. X and Y are sides of the triangle, Z is the hypotenuse. Angles A, B and C are unknown.
Fig 1. [image description]
  1. If X = 6 and Y = 12, then Z =         . Calculate to the nearest hundredth.
  2. If Z = 25 and Y = 15, the X =         . Calculate to the nearest hundredth.
  3. If angle A = 10° and Y = 150, then Z =         . Calculate to the nearest hundredth.
  4. If angle A = 45 and Z = 200, then X =         . Calculate to the nearest hundredth.
  5. If X = 100 and Y = 33, then angle B =         °. Calculate to the nearest hundredth.
  6. If Angle A is 22.5° and X = 45, then angle C =         °. Calculate to the nearest hundredth.

Answers

  1. 13.42
  2. 20
  3. 863.82
  4. 141.42
  5. 71.74 (degrees)
  6. 90 (degrees)

Practice Test

  1. A swimming pool that holds a maximum of 5000 cubic feet will hold         imperial gallons. Calculate the value to the nearest hundredth.
  2. A gas tank of a car has a volume of 3.62 cubic feet. In order to fill up the tank, it would take         US gallons. Calculate the value to the nearest hundredth.
  3. You are given a 5 US gallon pail to fill with water.  If you fill it up to the top it will weigh         pounds. Calculate the value to the nearest hundredth.
  4. 4 Litres of water weighs 4 pounds. True or false?
  5. A 4 litre milk carton has         imperial gallons. Calculate the value to the nearest hundredth.
  6. “Force over a defined area” is the definition of:
    1. Pressure
    2. Force
    3. PSI
    4. Newton
  7. The pressure (in psi) at the base of a piping system filled with water with a height of 17 feet would be          psi. Calculate the value to the nearest hundredth.
  8. A piping system runs through three storeys of a building. Each story is 9 feet high. Water is filled to the top of the third storey. What is the total force at the base of the piping system given that the pipe has a diameter of 4 inches?Calculate the value to the nearest hundredth.
  9. The total force acting at the base of a swimming pool which measures 40 feet by 20 feet and haas a depth of 5 feet is          pounds. Calculate the value to the nearest hundredth.
  10. A piping system requires that a 6 inch gate valve be installed.  It is determined that the system pressure is 120 psi and this pressure will be applied to the gate valve when it is closed.  The total force acting on the gate valve is         pounds. Calculate the value to the nearest hundredth.
  11. A pipe is run at a grade of 1/8” /ft. The Pipe is run a distance of 324 feet. The total fall on the pipe is          inches. Calculate the value to the nearest hundredth.
  12. A storm drain runs 100 feet. The grade on the storm drain is 1/4″ /ft. The total fall of the storm drain is          inches. Calculate to the nearest inch.
  13. A 157 meter 6” drainage line required to slope at a grade of 1/100. The total fall of the line is          metres. Calculate the value to the nearest hundredth.
  14. A pipe runs a distance of 500 feet. During the run of the pipe it drops 3 feet. The grade of the pipe (expressed as a percent) is         %. Calculate to the nearest tenth.
  15. A branch in a drainage system is 8 feet long. The grade on the pipe is 2.08%. The total fall on the branch (in inches) is *1.99/2* inches. Calculate to the nearest hundredth.

Use the image of the triangle below to answer the following questions:

A right triangle. X and Y are sides of the triangle, Z is the hypotenuse. Angles A, B and C are unknown.
Fig 2. [image description]
  1. If X = 6 and Y = 12, then Z =         . Calculate to the nearest hundredth.
  2. If Z = 25 and Y = 15, the X =         . Calculate to the nearest hundredth.
  3. If angle A = 10° and Y = 150, then Z =         . Calculate to the nearest hundredth.
  4. If angle A = 45 and Z = 200, then X =         . Calculate to the nearest hundredth.
  5. If X = 100 and Y = 33, then angle B =         °. Calculate to the nearest hundredth.
  6. If Angle A is 22.5° and X = 45, then angle C =         °. Calculate to the nearest hundredth.

Answers

  1. 31,200 (imperial gallons)
  2. 27.10 or 27.08 (US gallons)
  3. 41.65 (pounds)
  4. False
  5. 0.88 (imperial gallons)
  6. Pressure
  7. 75.78 (psi)
  8. 146.90 pounds
  9. 249,408 (pounds)
  10. 3392.93 or 3393 (pounds)
  11. 40.5 (inches)
  12. 25 (inches)
  13. 1.57 (metres)
  14. 0.6 (%)
  15. 1.99 or 2 (inches)
  16. 13.42
  17. 20
  18. 863.82
  19. 141.42
  20. 71.74 (degrees)
  21. 90 (degrees)

Image Descriptions

Figure 1 image description: A right triangle with angles A, B, and C and sides X, Y, and Z. Angle A is opposite side Y and adjacent to side X. Angle B is opposite side X and adjacent to side Y. Side Z is the hypotenuse. Angle C is opposite side Z. [Return to figure 1]

Figure 2 image description: A right triangle with angles A, B, and C and sides X, Y, and Z. Angle A is opposite side Y and adjacent to side X. Angle B is opposite side X and adjacent to side Y. Side Z is the hypotenuse. Angle C is opposite side Z. [Return to figure 2]

License

Icon for the Creative Commons Attribution 4.0 International License

Math for Trades: Volume 3 Copyright © 2022 by Mark Overgaard and Chad Flinn is licensed under a Creative Commons Attribution 4.0 International License, except where otherwise noted.

Share This Book