1.2: How to Use the Features of this Book to Your Advantage
How to Solve a Math Problem
Do you find it difficult to start a math problem? What do you do first? What is the next step? The reason this is challenging to some students is that they have never been shown problem-solving techniques. This textbook extensively uses an author-created design called the PUPP model in all guided examples. Successfully working through a problem involves four steps.
PUPP
- Plan,
- Understand
- What You Already Know
- How Will You get There
- Perform, and
- Present
The figure below shows the PUPP model at work. This model provides a structured problem-solving approach that will aid you not only in mathematics but in any problem-solving situation you may encounter.
Example: Straightforward Interest Rate Calculation—FV and PV Known
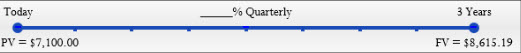
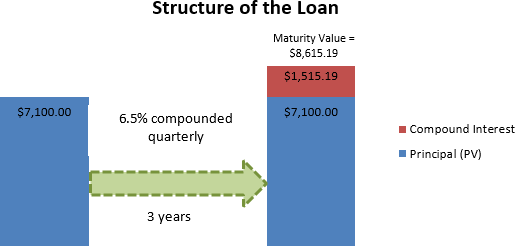
When Sandra borrowed $7,100 from Sanchez, she agreed to reimburse him $8,615.19 three years from now including interest compounded quarterly. What interest rate is being charged?
Plan
Find the nominal quarterly compounded rate of interest (IY).
What You Already Know (Understand)
Step 1:
- The present value, future value, term, and compounding are known, as illustrated in the timeline.
- CY = quarterly = 4
- Term = 3 years
How You Will Get There (Understand)
Step 2: Calculate N using Formula 9.2.
Step 3: Substitute into Formula 9.3 and rearrange for i. Step 4: Substitute into Formula 9.1 and rearrange for IY.
Perform
Step 2:
- N = 4 × 3 = 12
Step 3:
- $8,615.10 = $7,100(1 + i)12
- 1.213394 = (1 + i)12
- 1
- 1.21339412 = 1 + 𝑖𝑖0.016249 = i
Step 4:
- 0.016249 = IY IY = 0.064996 = 0.065 or 6.5%
- 4
Calculator Instructions
![]()
Present
Sanchez is charging an interest rate of 6.5% compounded quarterly on the loan to Sandra.
Using the PUPP Model
To understand each of the steps in the PUPP model, let’s plan a vacation.
Plan.
If you are heading out on vacation, the first thing you want to know is where you are going! Otherwise, how would you get there? In mathematics, you need to figure out what variable you are solving for before you can proceed. This step focuses your problem solving on the end goal. Many students mistakenly rush through this step and start punching numbers on a calculator or plugging numbers into formulas without understanding what it is they are doing. Spend plenty of time on this step, because the remaining three steps do not help at all if you solve for the wrong variable!
Understand – What You Already Know & How Will You get There.
In planning your vacation, once you figure out where you want to go you need to gather information about your destination. There are things you already know, but there are also things that you do not know, so you need a plan to figure these out. This step of the PUPP model helps your problem solving in two ways:
-
- What You Already KnowFirst, you need to assess what information has already been provided to you. You need to assign values to variables. You may need to draw diagrams to understand how all the numbers fit together.
- How Will You get ThereSecond, you create a plan by which you will solve your problem. Your roadmap identifies how you go from Point A (some variable is unknown) to Point B (knowing what the variable is).
Perform.
Once your vacation is planned, all that is left is to go on vacation! In other words, execute your plan. This step involves all of the mechanics and computations of your roadmap that you developed in the previous step.
Present.
After you return from vacation, you tell everyone about what you just did. In mathematics, this means you must explain solutions in the context of the question asked. If you just calculated x = $700, what exactly does that represent and how should it be interpreted? Calculating a solution without understanding it is not good enough. A further benefit of this last step is that you can detect errors more easily when you have to explain the solution. For example, read this presentation statement: “If an investor puts $1,000 into a savings account that earns 10% interest, I have calculated thatafter one year the investor has $700 in her savings account.” Did that make sense? If an account is earning interest, the balance should have become bigger, not smaller! Clearly, the solution of x = $700 must be an error!
Once you repeatedly practise this model and ingrain it in your thinking process, you have a structured approach to solving not just math problems but any life problem. When you are first learning the PUPP model you will need to write out every step of the model. As you keep practising, you’ll find that the model becomes a way of thinking. You can never skip one of the steps, but you won’t have to write them all out anymore because you’ll execute some steps with rapid-fire thought!
Understanding Formulas
You can’t avoid formulas—this is math, after all. What helps, though, is to understand what the formulas do and how each component comes together to produce the solution. If you can understand, you do not need to memorize! This book lays formulas out visually, clearly labelling all symbols. In addition, each component is fully explained (see below). You are not required to cross-reference paragraph-style explanations to formulas, as the visual layout makes it all clear. The goal is for you to understand what exactly the formula does and how, so that you understand enough to recognize when solutions make sense or might be wrong.
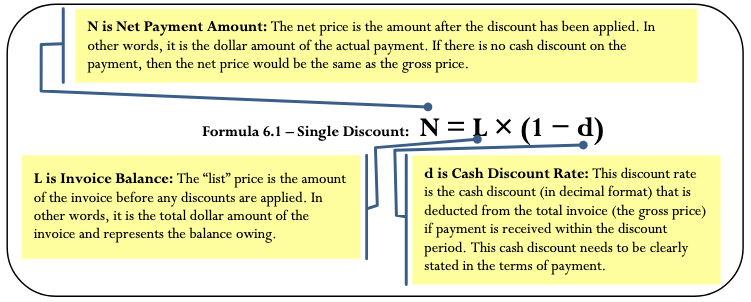
Additionally, you will not find any of those archaic algebraic symbols in this book. Representative symbols make the algebra easier to remember and understand. Those ancient mathematicians just loved to speak Greek (literally!), but this text speaks modern-day English and understands that we have technology here in the twenty-first century! We’ll use symbols like N for net price, L for list price, P for profit, and E for expenses, letters that actually remind you of the variable they stand for!
Does Anyone Actually Do Any of This in the Real World?
As you read through the guided examples and do your homework, pause to consider how the question applies to you. Does it demonstrate a business application you can use to be a smarter business professional? How can you extend the question to similar situations? This textbook shows you examples from both business and personal situations that you either have already encountered or probably will encounter in the future. Remember: Math is all around you!
- Business. Why do business programs require a business math course in the first term or two? How is it relevant to your future? This textbook provides numerous examples of applications in marketing, finance, economics, accounting, and more. Once you complete this textbook, you should see clearly how business mathematics is relevant and important to your chosen future career.
- Personal. You will see companies and products from your everyday life. Real-world situations show you how to put a few extra dollars in your pocket by making smart mathematical choices. What if you could save $100 per month simply by making smarter choices? Over 40 years at a very conservative rate of interest, that is approximately $100,000 in your pocket. You will also find life lessons. Are you ready to start planning your RRSP? Would you like to know the basics on how to do this? If you are thinking of buying a home, do you know how the numbers work on your mortgage? To learn how to buy a car at the lowest cost, read on!
Paths To Success
This feature is designed to make you feel like part of the “in” crowd by letting you take advantage of shortcuts or tricks of the trade, such as some easy way to remember a particular technique or formula. It helps you see how some of the math could be made a little simpler or performed in a different way.
Things to Watch Out For
Awareness of common pitfalls and sources of error in mathematics reduces your chances of making a mistake. A reminder also helps when important concepts are about to be used, reused, or combined in a novel way. It gives you a “heads up” when needed.
Give it Some Thought
Mastering business math requires two key skills:
- Executing the required techniques and calculations
- Understanding and successfully integrating various mathematical concepts. (For example, when a variable changes, can you estimate how this affects the final result?)
The second skill is much harder to acquire than the first. The Give It Some Thought section, poses scenarios and questions for which no calculations are required. You need to visualize and conceptualize various mathematical theorems. Ultimately, you perform a self-test to see if you are “getting it.”
Practice Questions
At the end of almost every section of this book you will find approximately 18 practice questions covering the concepts specifically introduced in that section, with final solutions listed to all questions at the end of this textbook. These questions are divided into three categories to help develop your mathematical skills and assess your abilities:
- Mechanics.
This group of questions focuses on fundamental mathematical skills and formula usage. They are your first taste of using the section formulas and working with the mathematical concepts at an introductory level. Successful completion of the Mechanics section means that you:- Have at least a basic understanding of the variables at play,
- Show rudimentary application of concepts, and
- Can calculate solutions using the formulas.
- Applications.
Applying your knowledge. These questions are more typical of business math course expectations (check with your instructor). The key difference from the Mechanics section is that you must now execute your problem-solving skills. These questions require you to determine the unknown variable and figure out how the various pieces of the puzzle come together in the solution. Successful completion of these questions means that you- Understand concepts at a satisfactory level,
- Can problem solve typical business math applications, and
- Can successfully integrate concepts at an acceptable performance level.
- Challenge, Critical Thinking, and Other Applications.
These questions put your knowledge and understanding to the test. The difficulty bar is set high, as these questions commonly require multistep solutions with advanced problem-solving techniques. As well, success might require you to integrate formulas, concepts, or procedures in novel ways. You will be challenged! Successful completion of these questions means that you- Understand concepts at an above-average level,
- Can problem solve in difficult situations, and
- Can successfully integrate concepts at a higher level of thinking.
At this point, I hope that you can see that all the features of this textbook are built for you! They are here to help you learn, understand, and perform math. This book gives you every opportunity to succeed!
Now that you know what business mathematics is and why it is important to you, let’s get going! Remember to take advantage of all the features of this textbook along the way.

