2.3 Percentages
How Does It All Relate?
Your class just wrote its first math quiz. You got 13 out of 19 questions correct, or 13/19. In speaking with your friends Sandhu and Illija, who are in other classes, you find out that they also wrote math quizzes; however, theirs were different.
Sandhu scored 16 out of 23, or 16/23, while Illija got 11 out of 16, or 11/16. Who achieved the highest grade? Who had the lowest? The answers are not readily apparent, because fractions are difficult to compare.
Now express your grades in percentages rather than fractions. You scored 68%, Sandhu scored 70%, and Illija scored 69%. Notice you can easily answer the questions now. The advantage of percentages is that they facilitate comparison and comprehension.
Converting Decimals to Percentages
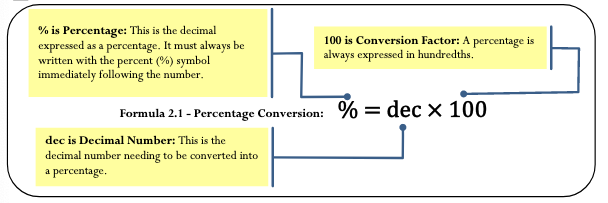
A percentage is a part of a whole expressed in hundredths. In other words, it is a value out of 100. For example, 93% means 93 out of 100, or 93/100 .
Formula 2.1
How It Works
Assume you want to convert the decimal number 0.0875 into a percentage. This number represents the dec variable in the formula. Substitute into Formula 2.1:
% = 0.0875 × 100 = 8.75%
Important Notes
You can also solve this formula for the decimal number. To convert any percentage back into its decimal form, you need to perform a mathematical opposite. Since a percentage is a result of multiplying by 100, the mathematical opposite is achieved by dividing by 100. Therefore, to convert 81% back into decimal form, you take 81% ÷ 100 = 0.81.
Things To Watch Out For
Your Texas Instruments BAII Plus calculator has a % key that can be used to convert any percentage number into its decimal format. For example, if you press 81 and then %, your calculator displays 0.81.
While this function works well when dealing with a single percentage, it causes problems when your math problem involves multiple percentages. For example, try keying 4% + 3% = into the calculator using the % key. This should be the same as 0.04 + 0.03 = 0.07. Notice, however, that your calculator has 0.0412 on the display.
Why is this? As a business calculator, you BAII Plus is programmed to take portions of a whole. When you key 3% into the calculator, it takes 3% of the first number keyed in, which was 4%. As a formula, the calculator sees 4% + (3% × 4%). This works out to 0.04 + 0.0012 = 0.0412.
To prevent this from happening, your best course of action is not to use the % key on your calculator. It is best to key all percentages as decimal numbers whenever possible, thus eliminating any chance that the % key takes a portion of your whole. Throughout this textbook, all percentages are converted to decimals before calculations take place.
Paths To Success
When working with percentages, you can use some tricks for remembering the formula and moving the decimal point.
Remembering the Formula
When an equation involves only multiplication of all terms on one side with an isolated solution on the other side, use a mnemonic called the triangle technique. In this technique, draw a triangle with a horizontal line through its middle. Above the line goes the solution, and below the line, separated by vertical lines, goes each of the terms involved in the multiplication. The figure to the right shows how Formula 2.1 would be drawn using the triangle technique.
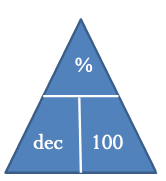 To use this triangle, identify the unknown variable, which you then calculate from the other variables in the triangle:
To use this triangle, identify the unknown variable, which you then calculate from the other variables in the triangle:
- Anything on the same line gets multiplied together. If solving for %, then the other variables are on the same line and multiplied as dec × 100.
- Any pair of items with one above the other is treated like a fraction and divided. If solving for dec, then the other variables are above/below each other and are divided as %/100 .
Moving the Decimal
Another easy way to work with percentages is to remember that multiplying or dividing by 100 moves the decimal over two places.
- If you are multiplying by 100, the decimal position moves two positions to the right.
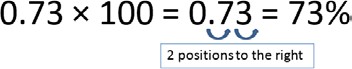
- If you are dividing by 100, the decimal position moves two positions to the left (see Figure 2.5).
![]()
Example 2.3A: Working with Percentages
- Convert (a) and (b) into percentages.
- Convert (c) back into decimal format.
| a. |
|
| b. | 1.3187 |
| c. | 12.399 % |
Plan
For questions (a) and (b), you need to convert these into percentage format.
For question (c), you need to convert it back to decimal format.
What You Already Know
- This is a fraction to be converted into a decimal, or dec.
- This is dec.
- This is %.
How You Will Get There
- Convert the fraction into a decimal to have dec.
Then apply Formula 2.1:
% = dec × 100 to get the percentage.
- As this term is already in decimal format, apply Formula 2.1: % = dec × 100
to get the percentage. - This term is already in percentage format. Using the triangle technique, calculate the decimal number through dec = %/100.
Perform
| A. |
|
|
|
| B. |
|
|
|
| C. |
|
Present
In percentage format, the first two numbers are 37.5% and 131.87%. In decimal format, the last number is 0.12399.
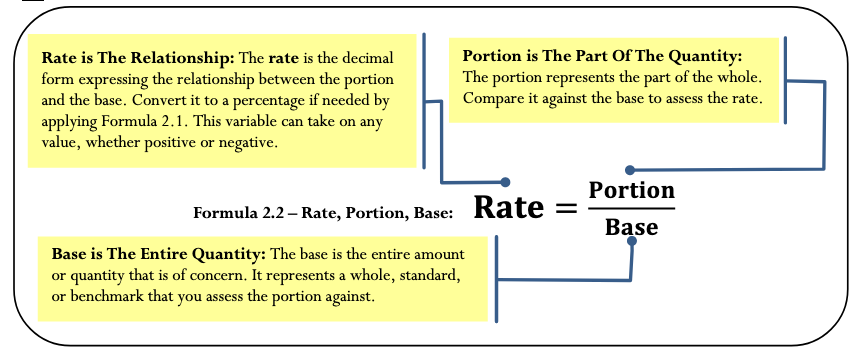
Rate, Portion, Base
In your personal life and career, you will often need to either calculate or compare various quantities involving fractions. For example, if your income is $3,000 per month and you can’t spend more than 30% on housing, what is your maximum housing dollar amount? Or perhaps your manager tells you that this year’s sales of $1,487,003 are 102% of last year’s sales. What were your sales last year?
Formula 2.2
How It Works
Assume that your company has set a budget of $1,000,000. This is the entire amount of the budget and represents your base. Your department gets $430,000 of the budget—this is your department’s part of the whole and represents the portion. You want to know the relationship between your budget and the company’s budget. In other words, you are looking for the rate.
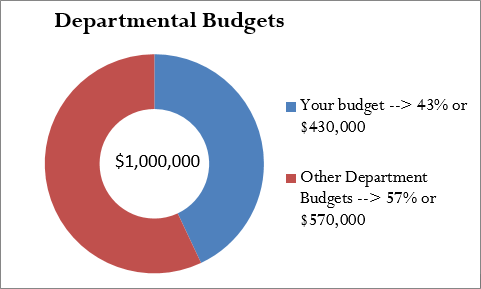
- Apply Formula 2.2, where rate = $430,000 / $1,000,000.
- Your budget is 0.43, or 43%, of the company’s budget.
Important Notes
There are three parts to this formula. Mistakes commonly occur through incorrect assignment of a quantity to its associated variable. The table below provides some tips and clue words to help you make the correct assignment.
|
Variable |
Key Words |
Example |
|
Base |
of |
If your department can spend 43% of the company’s total budget of $1,000,000, what is your maximum departmental spending? |
|
Portion |
is, are |
If your department can spend 43% of the company’s total budget of $1,000,000, what is your maximum departmental spending? |
|
Rate |
%, percent, rate |
If your department can spend 43% of the company’s total budget of $1,000,000, what is your maximum departmental spending? |
Things To Watch Out For
In resolving the rate, you must express all numbers in the same units—you cannot have apples and oranges in the same sequence of calculations. In the above example, both the company’s budget and the departmental budget are in the units of dollars. Alternatively, you would not be able to calculate the rate if you had a base expressed in kilometres and a portion expressed in metres. Before you perform the rate calculation, express both in kilometres or both in metres.
Paths To Success
 Formula 2.2 is another formula you can use the triangle technique for. You do not need to memorize multiple versions of the formula for each of the variables. The triangle appears to the right.
Formula 2.2 is another formula you can use the triangle technique for. You do not need to memorize multiple versions of the formula for each of the variables. The triangle appears to the right.
Be very careful when performing operations involving rates, particularly in summing or averaging rates.
Summing Rates
Summing rates requires each rate to be a part of the same whole or base. If Bob has 5% of the kilometres travelled and Sheila has 6% of the oranges, these are not part of the same whole and cannot be added. If you did, what does the 11% represent? The result has no interpretation. However, if there are 100 oranges of which Bob has 5% and Sheila has 6%, the rates can be added and you can say that in total they have 11% of the oranges.
Averaging Rates
Simple averaging of rates requires each rate to be a measure of the same variable with the same base. If 36% of your customers are female and 54% have high income, the average of 45% is meaningless since each rate measures a different variable. Recall that earlier in this chapter you achieved 68% on your test and Sandhu scored 70%. However, your test involved 19 questions and Sandhu’s involved 23 questions. These rates also cannot be simply averaged to 69% on the reasoning that (68% + 70%) / 2 = 69%, since the bases are not the same. When two variables measure the same characteristic but have different bases (such as the math quizzes), you must use a weighted-average technique.
When can you average rates? Hypothetically, assume Sandhu achieved his 70% by writing the same test with 19 questions. Since both rates measure the same variable and have the same base, the simple average of 69% is now calculable.
Give It Some Thought
Consider the following situations and select the best answer without performing any calculations.
- If the rate is 0.25%, in comparison to the base the portion is
-
- just a little bit smaller than the base.
- a lot smaller than the base.
- just a little bit bigger than the base.
- a lot bigger than the base.
- If the portion is $44,931 and the base is $30,000, the rate is
-
- smaller than 100%.
- equal to 100%.
- larger than 100% but less than 200%.
- larger than 200%.
- If the rate is 75% and the portion is $50,000, the base is
-
- smaller than $50,000.
- larger than $50,000.
- the same as the portion and equal to $50,000.
Solutions
1. b (0.25% is 0.0025, resulting in a very small portion)
2. c (the portion is larger than the base, but not twice as large)
3. b (the portion represents 75% of the base, meaning the base must be larger
Example 2.3B: Rate, Portion, Base
Solve for the unknown in the following three scenarios.
- If your total income is $3,000 per month and you can’t spend more than 30% on housing, what is the maximum amount of your total income that can be spent on housing?
- Your manager tells you that 2014 sales are 102% of 2013 sales. The sales for 2014 are $1,487,003. What were the sales in 2013?
- In Calgary, total commercial real estate sales in the first quarter of 2008 were $1.28 billion. The industrial, commercial, and institutional (ICI) land sector in Calgary had sales of $409.6 million. What percentage of commercial real estate sales is accounted for by the ICI land sector?
Plan
- You are looking for the maximum amount of your income that can be spent on housing.
- You need to figure out the sales for 2013.
- You must determine the percentage of commercial real estate sales accounted for by the ICI land sector in Calgary.
What You Already Know
- Look for key words in the question: “what is the maximum amount” and “of your total income.” The total income is the base, and the maximum amount is the portion.
Base = $3,000Rate = 30% Portion = maximum amount - Look for key words in the question: “sales for 2014 are $1,487,003” and “of 2013 sales.” The 2014 sales is the portion, and the 2013 sales is the base.
Portion = $1,487,003 Rate = 102% Base = 2013 sales - Look for key words in the question: “of commercial real estate sales” and “are accounted for by the ICI land sector.” The commercial real estate sales are the base, and the ICI land sector sales are the portion.
Base=$1.28 billion Portion=$409.6 million Rate=percentage
How You Will Get There
- Apply Formula 2.2, but rearrange using the triangle technique to have:
Portion = Rate × Base. - Apply Formula 2.2, but rearrange using the triangle technique to have:
Base=Portion/Rate. - Apply Formula 2.2:
Rate=Portion/Base.
Perform
| 1. |
|
| 2. |
|
| 3. |
|
Present
- The maximum you can spend on housing is $900 per month.
- 2013 sales were $1,457,846.08.
- The ICI land sector accounted for 32% of commercial real estate sales in Calgary for the first quarter of 2008.
Section 2.3 Exercises
Mechanics
- Convert the following decimals into percentages.
a. 0.4638b. 3.1579c. 0.000138d. 0.015 - Convert the following fractions into percentages.
![Rendered by QuickLaTeX.com \[ a. \frac {3}{8} \]](https://pressbooks.nscc.ca/app/uploads/quicklatex/quicklatex.com-4a0a08a839e9ea239054bd9b63e7037d_l3.png)
![Rendered by QuickLaTeX.com \[ b. \frac {17}{32} \]](https://pressbooks.nscc.ca/app/uploads/quicklatex/quicklatex.com-c1018eed18e5222e0ab20e0e9d87c5f0_l3.png)
![Rendered by QuickLaTeX.com \[ c. \frac {42}{12} \]](https://pressbooks.nscc.ca/app/uploads/quicklatex/quicklatex.com-5fcdd7178956ad2a422fb4b9fdb787e9_l3.png)
![Rendered by QuickLaTeX.com \[ d. 2 \frac {4}{5} \]](https://pressbooks.nscc.ca/app/uploads/quicklatex/quicklatex.com-6b960bcc0a81e593080280bf62a30ec3_l3.png)
- Convert the following fractions into percentages. Round to four decimals or express in repeating decimal format as needed.
![Rendered by QuickLaTeX.com \[ a. \frac {46}{12} \]](https://pressbooks.nscc.ca/app/uploads/quicklatex/quicklatex.com-84dce0180ece2cf8677ca0c524f887dc_l3.png)
![Rendered by QuickLaTeX.com \[ b. \frac {2}{9} \]](https://pressbooks.nscc.ca/app/uploads/quicklatex/quicklatex.com-cb588d8424003fbdaee743535ea37a3f_l3.png)
![Rendered by QuickLaTeX.com \[ c. \frac {3}{11} \]](https://pressbooks.nscc.ca/app/uploads/quicklatex/quicklatex.com-3ebb1833c681365f32245797bb2f2546_l3.png)
![Rendered by QuickLaTeX.com \[ d. \frac {48}{93} \]](https://pressbooks.nscc.ca/app/uploads/quicklatex/quicklatex.com-dd8b6046e5df9460381b2a07262d634a_l3.png)
- Convert the following percentages into decimal form.
a. 15.3% b. 0.03% c. 153.987% d. 14.0005% - What percentage of $40,000 is $27,000?
- What is ½% of $500,000?
- $0.15 is 4,900% of what number?
Applications
- In February 2009, 14,676 mortgages were in arrears in Canada, which represented 0.38% of all mortgages. How many total mortgages were in the Canadian market at that time?
- In 2009, medical experts predicted that one out of two Manitobans would contract some form of the H1N1 virus. If the population of Manitoba in 2009 was 1,217,200, how many Manitobans were predicted to become ill?
- In August 2004, Google Inc. offered its stocks to the public at $85 per share. In October 2007, the share price had climbed to $700.04. Express the 2007 share price as a percentage of the 2004 price.
- During Michael Jordan’s NBA career (1984–2003), he averaged a free throw completion percentage of 83.5% in regular season play. If Jordan threw 8,772 free throws in his career, how many completed free throws did he make?
- If total advertising expenditures on television advertising declined 4.1% to $141.7 billion in the current year, how much was spent on television advertising in the previous year? Round your answer to one decimal.
- If the new minimum wage of $8.75 per hour is 102.9412% of the old minimum wage, what was the old minimum wage?
- A machine can produce 2,500 products per hour. If 37 of those products were defective, what is the defect rate per hour for the machine?
Challenge, Critical Thinking, & Other Applications
- In 2011, Manitoba progressive income tax rates were 10.8% on the first $31,000, 12.75% on the next $36,000, and 17.4% on any additional income. If your gross taxable earnings for the year were $85,000, what percentage of your earnings did you pay in taxes?
- In 2011, the maximum amount that you could have contributed to your RRSP (Registered Retirement Savings Plan) was the lesser of $22,450 or 18% of your earned income from the previous year. How much income do you need to claim a $22,450 contribution in 2011?
- Maria, a sales representative for a large consumer goods company, is paid 3% of the total profits earned by her company. Her company averages 10% profit on sales. If Maria’s total income for the year was $60,000, what total sales did her company realize?
- A house was purchased six years ago for $214,000. Today it lists at a price that is 159.8131% of the original purchase price. In dollars, how much has the price of the house increased over the six years?
- An investor buys 1,000 shares of WestJet Airlines at $10.30 per share. A few months later, the investor sells the shares when their value hits 120% of the original share price. What is the price of a WestJet share when the investor sells these shares? How much money did the investor make?
- A Honda Insight has fuel economy of 3.2 litres consumed per 100 kilometres driven. It has a fuel tank capacity of 40 litres. A Toyota Prius is rated at 4.2 L per 100 km driven. It has a fuel tank capacity of 45 L. What percentage is the total distance driveable (rounded to the nearest kilometre before calculating) of a Honda Insight compared to that of a Toyota Prius?

