11.10 Markup

NSCC; Lumen Learning

11.10 Markup

Markup

As you wait in line to purchase your Iced Caramel Macchiato at Starbucks, you look at the pricing menu and think that $4.99 seems like an awful lot of money for a frozen coffee beverage. Clearly, the coffee itself doesn’t cost anywhere near that much. But then gazing around the café, you notice the carefully applied colour scheme, the comfortable seating, the high-end machinery behind the counter, and a seemingly well-trained barista who answers customer questions knowledgeably. Where did the money to pay for all of this come from? You smile as you realize your $4.99 pays not just for the macchiato, but for everything else that comes with it.

The process of taking a product’s cost and increasing it by some amount to arrive at a selling price is called markup. This process is critical to business success because every business must ensure that it does not lose money when it makes a sale. From the consumer perspective, the concept of markup helps you make sense of the prices that businesses charge for their products or services. This in turn helps you to judge how reasonable some prices are (and hopefully to find better deals).

The Components in a Selling Price

Before you learn to calculate markup, you first have to understand the various components of a selling price. Then, in the next section, markup and its various methods of calculation will become much clearer.

When your business acquires merchandise for resale, this is a monetary outlay representing a cost. When you then resell the product, the price you charge must recover more than just the product cost. You must also recover all the selling and operating expenses associated with the product. Ultimately, you also need to make some money, or profit, as a result of the whole process.

Most people think that marking up a product must be a fairly complex process. It is not. Formula 1.5 illustrates the relationship between the three components of cost, expenses, and profits in calculating the selling price.

Formula 1.5 – The Selling Price of a Product

$S=C+E+P$

S is Selling Price: Once you calculate what the business paid for the product (cost), the bills it needs to cover (expenses), and how much money it needs to earn (profit), you arrive at a selling price by summing the three components.

C is Cost: The cost is the amount of money that the business must pay to purchase or manufacture the product. If manufactured, the cost represents all costs incurred to make the product. If purchased, this number results from applying an appropriate discount formula. There is a list price from which the business will deduct discounts to arrive at the net price. The net price paid for the product equals the cost of the product. If a business purchases or manufactures a product for $10 then it must sell the product for at least $10. Otherwise, it fails to recover what was paid to acquire or make the product in the first place—a path to sheer disaster!

E is Expenses: Expenses are the financial outlays involved in selling the product. Beyond just purchasing the product, the business has many more bills to pay, including wages, taxes, leases, equipment, electronics, insurance, utilities, fixtures, décor, and many more. These expenses must be recovered and may be calculated as:

A fixed dollar amount per unit.
A percentage of the product cost. For example, if a business forecasts total merchandise costs of $100,000 for the coming year and total business expenses of $50,000, then it may set a general guideline of adding 50% ($50,000 ÷ $100,000) to the cost of a product to cover expenses.
A percentage of the product selling price based on a forecast of future sales. For example, if a business forecasts total sales of $250,000 and total business expenses of $50,000, then it may set a general guideline of adding 20% ($50,000 ÷ $250,000) of the selling price to the cost of a product to cover expenses.

P is Profit: Profit is the amount of money that remains after a business pays all of its costs and expenses. A business needs to add an amount above its costs and expenses to allow it to grow. If it adds too much profit, though, the product’s price will be too high, in which case the customer may refuse to purchase it. If it adds too little profit, the product’s price may be too low, in which case the customer may perceive the product as shoddy and once again refuse to purchase it. Many businesses set general guidelines on how much profit to add to various products. As with expenses, this profit may be expressed as:

A fixed dollar amount per unit.
A percentage of the product cost.
A percentage of the selling price.

How It Works

Follow these steps to solve pricing scenarios involving the three components:

Step 1: Four variables are involved in Formula 1.5. Identify the known variables. Note that you may have to calculate the product’s cost by applying the single or multiple discount formulas (Formulas 1.1 and 1.3, respectively). Pay careful attention to expenses and profits to capture how you calculate these amounts.

Step 2: Apply Formula 1.5 and solve for the unknown variable.

Assume a business pays a net price of $75 to acquire a product. Through analyzing its finances, the business estimates expenses at $25 per unit, and it figures it can add $50 in profit. Calculate the selling price.

Step 1: The net price paid for the product is the product cost. The known variables are:

$C = \char36 75$
$E = \char36 25$
$P = \char36 50$

Step 2: According to Formula 1.5, the unit selling price is:

$S=C+E+P=\char36 75+\char36 25+\char36 50=\char36 150$

In applying Formula 1.5 you must adhere to the basic rule of linear equations requiring all terms to be in the same unit. That is, you could use Formula 1.5 to solve for the selling price of an individual product, where the three components are the unit cost, unit expenses, and unit profit. When you add these, you calculate the unit selling price. Alternatively, you could use Formula 1.5 in an aggregate form where the three components are total cost, total expenses, and total profit. In this case, the selling price is a total selling price, which is more commonly known as total revenue. But you cannot mix individual components with aggregate components.

The most common mistake in working with pricing components occurs in the “Understand” portion of the PUPP model. It is critical to identify and label information correctly. You have to pay attention to details such as whether you are expressing the expenses in dollar format or as a percentage of either cost or selling price. Systematically work your way through the information provided piece by piece to ensure that you do not miss an important detail.

Give It Some Thought:

What three components make up a selling price? In what units are these components commonly expressed?
In what three ways are expenses and profits expressed?
What is the relationship between net price and cost?

Example 1.2A – Setting a Price on Fashion in Dollars

Mary’s Boutique purchases a dress for resale at a cost of $23.67. The owner determines that each dress must contribute $5.42 to the expenses of the store. The owner also wants this dress to earn $6.90 toward profit. What is the regular selling price for the dress?

Plan:
You are looking for the regular selling price for the dress, or $S$ .

Understand:
Step 1: The unit cost of the dress and the unit expense and the unit profit are all known:

$C = \char36 23.67$
$E = \char36 5.42$
$P = \char36 6.90$

Step 2: Apply Formula 1.5.

Perform:
Step 2:

$S=\char36 23.67+\char36 5.42+\char36 6.90=\char36 35.99$

Present:
Mary’s Boutique will set the regular price of the dress at $35.99.

Example 1.2B – Setting the Price Using Percentage of Cost

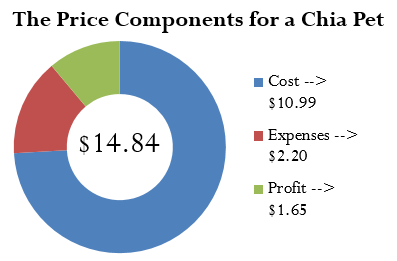
John’s Discount Store just completed a financial analysis. The company determined that expenses average 20% of the product cost and profit averages 15% of the product cost. John’s Discount Store purchases Chia Pets from its supplier for an MSRP of $19.99 less a trade discount of 45%. What will be the regular selling price for the Chia Pets?

Plan:
You are looking for the regular selling price for the Chia pets, or $S$ .

Understand:
Step 1: The list price, discount rate, expenses, and profit are known:

$L = \char36 19.99$
$d$ = 0.45
$E$ = 20% of cost, or 0.20C
$P$ = 15% of cost, or 0.15C

Although the cost of the Chia Pets is not directly known, you do know the MSRP (list price) and the trade discount. The cost is equal to the net price. Apply Formula 1.1.

Step 2: To calculate the selling price, apply Formula 1.5.

Perform:
Step 1:

$N=\char36 19.99\times(1-0.45)=\char36 19.99\times0.55=\char36 10.99=C$

Step 2:

$S=\char36 10.99+0.20C+0.15C$
$S=\char36 10.99+0.20(10.99)+0.15(\char36 10.99)$
$S=\char36 10.99+\char36 2.20+\char36 1.65=\char36 14.84$

Present:
John’s Discount Store will sell the Chia Pet for $14.84.

A circle diagram illustrating the price components for a Chia Pet. The circle represents the selling price of $14.84. The blue portion of the circle represents the cost ($10.99.) The red portion of the circle represents expenses ($2.20.) The green portion of the circle represents profit ($1.65.)

Example 1.2C – Setting the Price Using Percentage of Selling Price

Based on last year’s results, Benthal Appliance learned that its expenses average 30% of the regular selling price. It wants a 25% profit based on the selling price. If Benthal Appliance purchases a fridge for $1,200, what is the regular unit selling price?

Plan:
You are looking for the regular unit selling price for the fridge, or $S$ .

Understand:
Step 1: The cost, expenses, and profit for the fridge are known:

$E$ = 30% of $S$ , or $0.3S$
$P$ = 25% of $S$ , or $0.25S$
$C$ = $1,200.00

Step 2: Apply Formula 1.5.

Perform:
Step 2:

$S=\char36 1,200.00+0.3S+0.25S$
$S=\char36 1,200+0.55S$
$S-0.55S=\char36 1,200.00$
$0.45S=\char36 1,200.00$
$S=\char36 2,666.67$

Present
Benthal Appliance should set the regular selling price of the fridge at $2,666.67.

A circle diagram illustrating price components for a fridge. The circle represents the regular selling price ($2,666.67.) The blue portion of the circle represents cost ($1,200.00). The red portion of the circle represents expenses [0.3($2,666.67) = $800.00.] The green portion of the circle represents profit [0.25($2,666.67) = $666.67]

Example 1.2D – Using Selling Price to Figure Out the Cost

If a company knows that its profits are 15% of the selling price and expenses are 30% of cost, what is the cost of an MP3 player that has a regular selling price of $39.99?

Plan:
You are looking for the cost of the MP3 player, or $C$ .

Understand:
Step 1: The expenses, profits, and the regular unit selling price are as follows:

$S$ = $39.99
$P$ = 15% of $S$ , or $0.15S$
$E$ = 30% of cost, or $0.3C$

Step 2: Apply Formula 1.5, rearranging for $C$ .

Perform:
Step 2:

$\char36 39.99=C+0.3C+0.15(\char36 39.99)$
$\char36 39.99=1.3C+\char36 6.00$
$\char36 33.99=1.3C$
$\char36 26.15=C$

Present:
The cost of the MP3 Player is $26.15.

A circle diagram illustrating price components of an MP3 player. The circle represents the regular selling price ($39.99.) The blue portion of the circle represents cost ($26.15.) The red portion of the circle represents expenses [0.3($26.15) = $7.84.] The green portion of the circle represents profit [0.13($39.99) = $6.00.]

Example 1.2E – Determining Profitability

Peak of the Market considers setting the regular unit selling price of its strawberries at $3.99 per kilogram. If it purchases these strawberries from the farmer for $2.99 per kilogram and expenses average 40% of product cost, does Peak of the Market make any money?

Plan:
In asking whether Peak of the Market makes money, you are looking for the profit, or $P$ .

Understand:
Step 1: The cost, expenses, and proposed regular unit selling price for the strawberries are as follows:

$S$ = $3.99
$C$ = $2.99
$E$ = 40% of cost, or $0.4C$

Step 2: Apply Formula 1.5, rearranging for $P$ .

Perform:
Step 2:

$\char36 3.99=\char36 2.99+0.4(\char36 2.99)+P$
$\char36 3.99=\char36 4.19+P$
$-\char36 0.20=P$

Present:
The negative sign on the profit means that Peak of the Market would take a loss of $0.20 per kilogram if it sells the strawberries at $3.99. Unless Peak of the Market has a marketing reason or sound business strategy for doing this, the company should reconsider its pricing.

Calculating the Markup Dollars

Most companies sell more than one product, each of which has different price components with varying costs, expenses, and profits. Can you imagine trying to compare 50 different products, each with three different components? You would have to juggle 150 numbers! To make merchandising decisions more manageable and comparable, many companies combine expenses and profit together into a single quantity, either as a dollar amount or a percentage. This section focuses on the markup as a dollar amount.

One of the most basic ways a business simplifies its merchandising is by combining the dollar amounts of its expenses and profits together as expressed in Formula 1.6.

Formula 1.6 – Markup Amount

$M\char36 =E+P$

$M\char36$ is Markup Amount: Markup is taking the cost of a product and converting it into a selling price. The markup amount represents the dollar amount difference between the cost and the selling price.

$E$ is Expenses: The expenses associated with the product.

$P$ is Profit: The profit earned when the product sells.

Note that since the markup amount ( $M\char36$ ) represents the expenses ( $E$ ) and profit ( $P$ ) combined, you can substitute the variable for markup amount into Formula 1.5 to create Formula 1.7, which calculates the regular selling price.

Formula 1.7 – Selling Price Using Markup

$S=C+M\char36$

$S$ is Selling Price: The regular selling price of the product.

$C$ is Cost: The amount of money needed to acquire or manufacture the product. If the product is being acquired, the cost is the same amount as the net price paid.

$M\char36$ is Markup Amount: From Formula 1.6, this is the single number that represents the total of the expenses and profit.

How It Works

Follow these steps when you work with calculations involving the markup amount:

Step 1: You require three variables in either Formula 1.6 or Formula 1.7. At least two of the variables must be known. If the amounts are not directly provided, you may need to calculate these amounts by applying other discount or markup formulas.

Step 2: Solve either Formula 1.6 or Formula 1.7 for the unknown variable.

Recall from Example 1.2D that the MP3 player’s expenses are $7.84, the profit is $6.00, and the cost is $26.15. Calculate the markup amount and the selling price.

Step 1: The known variables are

$E$ = $7.84
$P$ = $6.00
$C$ = $26.15.

Step 2: According to Formula 1.6, the markup amount is the sum of the expenses and profit, or

$M\char36 =\char36 7.84+\char36 6.00=\char36 13.84$ .

Applying Formula 1.7, add the markup amount to the cost to arrive at the regular selling price, resulting in

$S=\char36 26.15+\char36 13.84=\char36 39.99$

You might have already noticed that many of the formulas in this chapter are interrelated. The same variables appear numerous times but in different ways. To help visualize the relationship between the various formulas and variables, many students have found it helpful to create a markup chart, as shown below.

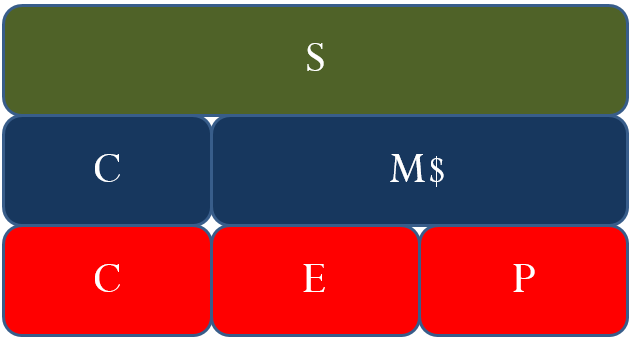
A chart demonstrating the relationships between Formulas 4.5, 4.6, and 4.7. (Described below.)

This chart demonstrates the relationships between Formulas 1.5, 1.6, and 1.7. It is evident that the selling price (the green line) consists of cost, expenses, and profit (the red line representing Formula 1.5); or it can consist of cost and the markup amount (the blue line representing Formula 1.7). The markup amount on the blue line consists of the expenses and profit on the red line (Formula 1.6).

Example 1.2F – Markup as a Dollar Amount

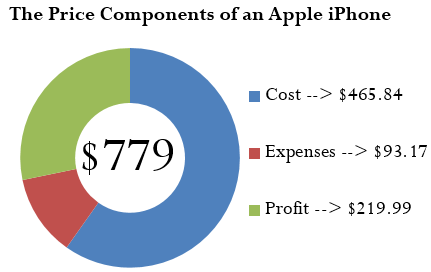
A cellular retail store purchases an iPhone with an MSRP of $779 less a trade discount of 35% and volume discount of 8%. The store sells the phone at the MSRP.
a. What is the markup amount?
b. If the store knows that its expenses are 20% of the cost, what is the store’s profit?

Plan:
First of all, you need to calculate the markup amount ( $M\char36$ ). You also want to find the store’s profit ( $P$ ).

Understand:
Step 1: The smartphone MSRP and the two discounts are known, along with the expenses and selling price:

$L$ = $270
$d_1$ = 0.35
$d_2$ = 0.08
$E$ = 20% of cost, or $0.2C$
$S$ = $779

Calculate the cost of the iPhone by applying Formula 1.3.

Step 2: Calculate the markup amount using Formula 1.7.

Calculate the profit by applying Formula 1.6, rearranging for $P$ .

Perform:
Step 1:

$N=\char36 779.00\times(1-0.35)\times(1-0.08)$
$N=\char36 779.00\times0.65\times0.92=\char36 465.84=C$

Step 2:

$\char36 779.00=\char36 465.84+M\char36$
$\char36 313.16=M\char36$

$\char36 313.16=0.2(\char36 465.84)+P$
$\char36 313.16=\char36 93.17+P$
$\char36 219.99=P$

Present:
The markup amount for the iPhone is $313.16. When the store sells the phone for $779.00, its profit is $219.99.

A circle diagram illustrating the price components of an Apple iPhone. The circle represents the selling price ($779.00). The blue portion of the circle represents the cost ($465.84). The red portion of the circle represents expenses ($93.17). The green portion of the circle represents profit ($219.99.)

Calculating the Markup Percent

It is important to understand markup in terms of the actual dollar amount; however, it is more common in business practice to calculate the markup as a percentage. There are three benefits to converting the markup dollar amount into a percentage:

Easy comparison of different products having vastly different price levels and costs, to help you see how each product contributes toward the financial success of the company. For example, if a chocolate bar has a 50¢ markup included in a selling price of $1, while a car has a $1,000 markup included in a selling price of $20,000, it is difficult to compare the profitability of these items. If these numbers were expressed as a percentage of the selling price such that the chocolate bar has a 50% markup and the car has a 5% markup, it is clear that more of every dollar sold for chocolate bars goes toward list profitability.
Simplified translation of costs into a regular selling price—a task that must be done for each product, making it helpful to have an easy formula, especially when a company carries hundreds, thousands, or even tens of thousands of products. For example, if all products are to be marked up by 50% of cost, an item with a $100 cost can be quickly converted into a selling price of $150.
An increased understanding of the relationship between costs, selling prices, and the list profitability for any given product. For example, if an item selling for $25 includes a markup on selling price of 40% (which is $10), then you can determine that the cost is 60% of the selling price ($15) and that $10 of every $25 item sold goes toward list profits.

You can translate the markup dollars into a percentage using two methods, which express the amount either as a percentage of cost or as a percentage of selling price:

Method 1: Markup as a Percentage of Cost.
This method expresses the markup rate using cost as the base. Many companies use this technique internally because most accounting is based on cost information. The result, known as the markup on cost percentage, allows a reseller to convert easily from a product’s cost to its regular unit selling price.
Method 2: Markup as a Percentage of Selling Price.
This method expresses the markup rate using the regular selling price as the base. Many other companies use this method, known as the markup on selling price percentage, since it allows for quick understanding of the portion of the selling price that remains after the cost of the product has been recovered. This percentage represents the list profits before the deduction of expenses and therefore is also referred to as the list profit margin.

The markup on cost percentage is expressed in Formula 1.8, while the markup on selling price percentage is expressed in Formula 1.9.

Formula 1.8 – Markup On Cost Percentage

$MoC\%=\frac{M\char36}{C}\times100$

$MoC\%$ is Markup on Cost Percentage: This is the percentage by which the cost of the product needs to be increased to arrive at the selling price for the product.

$M\char36$ is Markup Amount: The total dollars of the expenses and the profits; this total is the difference between the cost and the selling price.

$C$ is Cost: The amount of money needed to acquire or manufacture the product. If the product is being acquired, the cost is the same amount as the net price paid.

× 100 is Percent Conversion: The markup on cost is always a percentage.

Formula 1.9 – Markup On Selling Price Percentage

$MoS\%=\frac{M\char36}{S}\times100$

$MoS\%$ is Markup on Selling Price Percentage: This is the percentage of the selling price that remains available as list profits after the cost of the product is recovered.

$M$ is Markup Amount: The total dollars of the expenses and the profits; this total is the difference between the cost and the selling price.

$S$ is Selling Price: The regular selling price of the product.

× 100 is Percent Conversion: The markup on cost is always a percentage.

How It Works

Refer back to the steps in calculating markup. The steps you must follow for calculations involving markup percent are almost identical to those for working with markup dollars:

Step 1: Three variables are required in either Formula 1.8 or Formula 1.9. For either formula, at least two of the variables must be known. If the amounts are not directly provided, you may need to calculate these amounts by applying other discount or markup formulas.

Step 2: Solve either Formula 1.8 or Formula 1.9 for the unknown variable.

Continuing to work with Example 1.2D, recall that the cost of the MP3 player is $26.15, the markup amount is $13.84, and the selling price is $39.99. Calculate both markup percentages.

Step 1: The known variables are

$C$ = $26.15
$M\char36$ = $13.84
$S$ = $39.99.

Step 2: To calculate the markup on cost percentage, apply Formula 1.8:

$MoC\%=\frac{\char36 13.84}{\char36 26.15}\times100=52.9254\%$

In other words, you must add 52.9254% of the cost on top of the unit cost to arrive at the regular unit selling price of $39.99.

To calculate the markup on selling price percentage, apply Formula 1.9:

$MoS\%=\frac{\char36 13.84}{\char36 39.99}\times100=34.6087\%$

In other words, 34.6087% of the selling price represents list profits after the business recovers the $26.15 cost of the MP3 player.

Businesses are very focused on profitability. Your Texas Instruments BAII Plus calculator is programmed with the markup on selling price percentage. The function is located on the second shelf above the number three. To use this function, open the window by pressing 2nd 3. You can scroll between lines using your ↑ and ↓ arrows. There are three variables:

CST is the cost. Use the symbol $C$ .
SEL is the selling price. Use the symbol $S$ .
MAR is the markup on selling price percentage. Use the symbol $MoS\%$ .

As long as you know any two of the variables, you can solve for the third. Enter any two of the three variables (you need to press ENTER after each), making sure the window shows the output you are seeking, and press CPT.

Merchandising involves many variables. Nine formulas have been established so far, and a few more are yet to be introduced. Though you may feel bogged down by all of these formulas, just remember that you have encountered most of these merchandising concepts since you were very young and that you interact with retailers and pricing every day. This chapter merely formalizes calculations you already perform on a daily basis, whether at work or at home. The calculation of discounts is no different than going to Walmart and finding your favourite CD on sale. You know that when a business sells a product, it has to recoup the cost of the product, pay its bills, and make some money. And you have worked with percentages since elementary school.

Do not get stuck in the formulas. Think about the concept presented in the question. Change the scenario of the question and put it in the context of something more familiar. Ultimately, if you really have difficulties then look at the variables provided and cross-reference them to the merchandising formulas. Your goal is to find formulas in which only one variable is unknown. These formulas are solvable. Then ask yourself, “How does knowing that new variable help solve any other formula?”

You do not need to get frustrated. Just be systematic and relate the question to what you already know.

The triangle method simplifies rearranging both Formulas 1.8 and 1.9 to solve for other unknown variables as illustrated in the figure below.

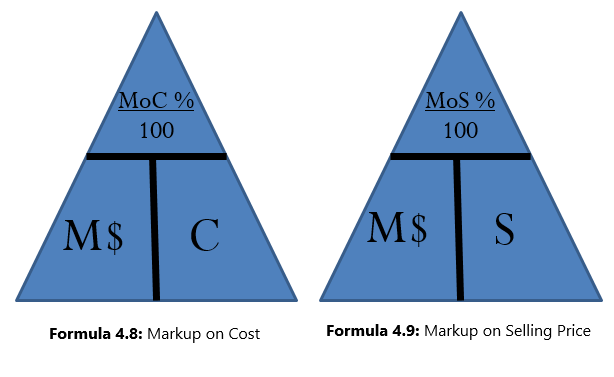
Two triangle diagrams illustrating how to rearrange formulas. The triangle on the left represents formula 4.8: markup on cost. The top sector of the triangle represents Moc%/100. The left sector represent M$. The right sector represents C. The triangle on the right represents formula 4.9: markup on selling price. The top sector of the triangle represents MoS%/100. The left sector represents M$. The right sector represents S.

Sometimes you need to convert the markup on cost percentage to a markup on selling price percentage, or vice versa. Two shortcuts allow you to convert easily from one to the other:

$MoC\%=\frac{MoS\%}{1-MoS\%}$

$MoS\%=\frac{MoC\%}{1+MoC\%}$

Notice that these formulas are very similar. How do you remember whether to add or subtract in the denominator? In normal business situations, the $MoC\%$ is always larger than the $MoS\%$ . Therefore, if you are converting one to the other you need to identify whether you want the percentage to become larger or smaller.

To calculate $MoC\%$ , you want a larger percentage. Therefore, make the denominator smaller by subtracting $MoS\%$ from 1.
To calculate $MoS\%$ , you want a smaller percentage. Therefore, make the denominator larger by adding $MoC\%$ to 1.

Give It Some Thought:

Answer the following true/false questions.

4. The markup on selling price percentage can be higher than 100%.

5. The markup dollar amount can be more than the selling price.

6. The markup on cost percentage can be higher than 100%.

7. The markup on cost percentage in most business situations is higher than the markup on selling price percentage.

8. If you know the markup on cost percentage and the cost, you can calculate a selling price.

9. If you know the markup on selling price percentage and the cost, you can calculate a selling price.

Example 1.2G – Markup as a Percentage

A large national retailer wants to price a Texas Instruments BAII Plus calculator at the MSRP of $39.99. The retailer can acquire the calculator for $17.23.
a. What is the markup on cost percentage?
b. What is the markup on selling price percentage?

Plan:
You have been asked to solve for the markup on cost percentage ( $MoC\%$ ) and the markup on selling price percentage ( $MoS\%$ ).

Understand:
Step 1: The regular unit selling price and the cost are provided:

$S$ = $39.99
$C$ = $17.23

You need the markup dollars. Apply Formula 1.7, rearranging for $M\char36$ .

Step 2: To calculate markup on cost percentage, apply Formula 1.8.

To calculate markup on selling price percentage, apply Formula 1.9.

Perform:
Calculator Instructions:

CST

SEL

MAR

17.23

39.99

Answer:
56.9142

Step 1:

$\char36 39.99=\char36 17.23+M\char36$
$\char36 22.76=M\char36$

Step 2:

$MoC\%=\frac{\char36 22.76}{\char36 17.23}\times100=132.0952\%$

$MoS\%=\frac{\char36 22.76}{\char36 39.99}\times100=56.9142\%$

Present:
The markup on cost percentage is 132.0952%. The markup on selling price percentage is 56.9142%.

The section on Markup is reproduced from Chapter 4.2 in Fundamentals of Business Mathematics by OER Lab licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

License

Icon for the Creative Commons Attribution 4.0 International License

Markup

The Components in a Selling Price

Calculating the Markup Dollars

Calculating the Markup Percent

License

Share This Book