14.8 Problems

Review Questions

1. What is the basic function of an integrator?
2. What is the basic function of a differentiator?
3. What is the function of the capacitor in the basic integrator and differentiator?
4. Why are capacitors used in favor of inductors?
5. What practical modifications need to be done to the basic integrator, and why?
6. What practical modifications need to be done to the basic differentiator, and why?
7. What are the negative side effects of the practical versus basic integrator and differentiator?
8. What is an analog computer, and what is it used for?
9. What are some of the advantages and disadvantages of the analog computer versus the digital computer?
10. How might integrators and differentiators be used as wave-shaping circuits?

Problems

Analysis Problems

1. Sketch the output waveform for the circuit of Figure 10.8.1 if the input is a 4 V peak square wave at 1 kHz.
   

   

2. Repeat Problem 1 if 𝑉𝑖𝑛(𝑡)=5sin2𝜋318𝑡 .
3. Repeat Problem 1 if 𝑉𝑖𝑛(𝑡)=20cos2𝜋10000𝑡 .
4. Using the circuit of Problem 1, if the input is a ramp with a slope of 10 V/s, find the output after 1 ms, 10 ms, and 4 s. Sketch the resulting wave.
5. Determine the low frequency gain for the circuit of Figure 10.8.2 .
   

   

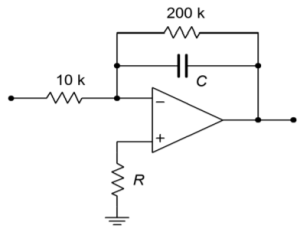
6. If 𝐶=33 nF in Figure 10.8.2 , determine 𝑉_𝑜𝑢𝑡if 𝑉_𝑖𝑛is a 200 mV peak sine wave at 50 kHz.
7. Repeat Problem 6 using a 200 mV peak square wave at 50 kHz.
8. Sketch the output of the circuit of Figure 10.2.7 with the input signal given in Figure 10.8.3 .
9. Assume that the input to the circuit of Figure 10.2.7 is 100 mV DC. Sketch the output waveform, and determine if it goes to saturation.
   

   

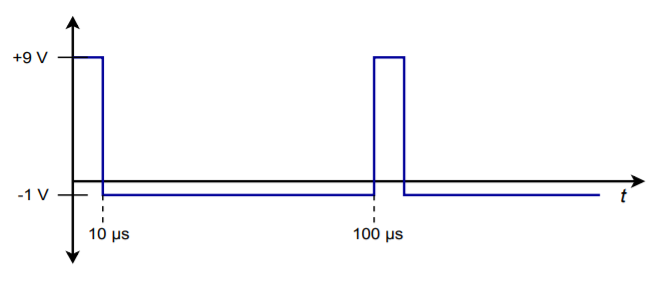
10. Sketch the output waveform for Figure 10.2.7, given an input of: 𝑉𝑖𝑛(𝑡)=0.5cos2𝜋9000𝑡 .
11. Given the circuit of Figure 10.8.4 , sketch the output waveform if the input is a 100 Hz, 1 V peak triangle wave.
    

    

12. Repeat Problem 11 if the input is a 2 V peak square wave at 500 Hz. Assume that the rise and fall times are 1 𝜇 s and are linear (vs. exponential).
13. Repeat Problem 11 for the following input: 𝑉𝑖𝑛(𝑡)=3cos2𝜋60𝑡 .
14. Repeat Problem 11 for the following input: 𝑉𝑖𝑛(𝑡)=0.5sin2𝜋1000𝑡 .
15. Repeat Problem 11 for the following input: 𝑉𝑖𝑛(𝑡)=10𝑡2 .
    

    

16. Given the input shown in Figure 10.8.5 , sketch the output of the circuit of Figure 10.19.
17. Given the input shown in Figure 10.8.5 , sketch the output of the circuit of Figure 10.19.
    

    

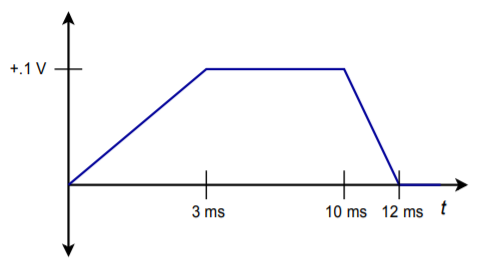
18. Sketch the output waveform for Figure 10.3.9, given an input of 𝑉𝑖𝑛(𝑡)=0.5cos2𝜋4000𝑡 .
19. Sketch the output waveform for Figure 10.3.9, if 𝑉_𝑖𝑛is a 300 mV peak triangle wave at 2500 Hz.

Design Problems

20. Given the circuit of Figure 10.8.1 ,
    1. Determine the value of 𝐶 required in order to yield an integration constant of −2000.
    2. Determine the required of value of 𝑅 for minimum integration offset error.
    3. Determine 𝑓𝑙𝑜𝑤 .
    4. Determine the 99% accuracy point.
21. Given the differentiator of Figure 10.8.7 , determine the value of 𝑅 that will set the differentiation constant to −103 .
    

    

22. Determine the value of 𝑅𝑖 such that the maximum gain is 20 for the circuit of Figure 10.8.7 . (Use the values of Problem 21.)
23. Determine the value of 𝐶𝑓 in Problem 22 such that noise above 5 kHz is attenuated.
24. Design an integrator to meet the following specifications: integration constant of −4500, 𝑓𝑙𝑜𝑤 no greater than 300 Hz, 𝑍𝑖𝑛 at least 6 k Ω , and DC gain no more than 32 dB.
25. Design a differentiator to meet the following specifications: differentiation constant of −1.2𝐸−4 , 𝑓ℎ𝑖𝑔ℎ at least 100 kHz, and a minimum 𝑍𝑖𝑛 of 50 Ω .

Challenge Problems

26. Sketch the output waveform for the circuit of Figure 10.2.7 if the input is the following damped sinusoid: 𝑉𝑖𝑛(𝑡)=3𝜀−200𝑡sin2𝜋1000𝑡 .
27. A 1.57 V peak, 500 Hz square wave may be written as the following infinite series: 𝑉𝑖𝑛(𝑡)=2∑∞𝑛=112𝑛−1sin2𝜋500(2𝑛−1)𝑡
    Using this as the input signal, determine the infinite series output Equation for the circuit of Figure 10.2.7.
28. Sketch the output for the preceding problem. Do this by graphically adding the first few terms of the output.
29. Remembering that the voltage across an inductor is equal to the inductance times the rate of change of current, determine the output Equation for the circuit of Figure 10.8.8 .
    

    

30. Repeat the preceding Problem for the circuit of Figure 10.8.9 .
    

    

31. A 2.47 V peak, 500 Hz triangle wave may be written as the following infinite series: 𝑉𝑖𝑛(𝑡)=2∑∞𝑛=11(2𝑛−1)2cos2𝜋500(2𝑛−1)𝑡
    Using this as the input signal, determine the infinite series output Equation for the circuit of Figure 10.3.9.
32. Sketch the output for the preceding problem. Do this by graphically adding the first few terms of the output.

Computer Simulation Problems

33. Simulate Problem 1 and determine the steady-state response.
34. Model Problem 8 using a simulator. Determine both the steady-state and initial outputs.
35. Simulate Problem 14. Determine the steady-state output.
36. Model Problem 22 using a simulator and determine the output signal.
37. Compare the resulting waveform produced by the circuit of Problem 8 using both the 741 op amp and the medium-speed LF411. Does the choice of op amp make a discernible difference in this application?